
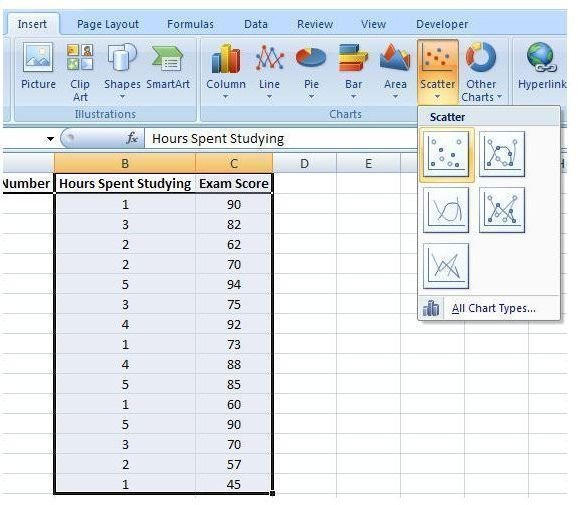
The observed relationships may be positive or negative, non-linear or linear, and strong or weak. The variable on the horizontal axis is sometimes referred to as an independent variable, whereas the variable on the vertical axis is referred to as a dependent variable. In these situations, we want to know what a successful vertical value estimate would be if we were given a specific horizontal value. Scatter plots are often used to identify correlational associations. In a scatter plot, the dots show not just the values of individual data points but also associations when the data is viewed as a whole. The primary purpose of a scatter plot is to observe and display relationships between two numeric variables. The relationship between more than two variables is not shown in this plot. It only depicts the quantitative representation of a quantitative change. The quantitative calculation of the relationship between the variables is not shown in a scatter diagram.

Scatter diagrams can't tell you how strong the association is. Causation means that any occurrence will affect the outcome. Correlation can never be mistaken for causation. When it comes to correlation, keep in mind that correlation does not imply that changes in one variable are responsible for changes in another. It can make determining relationships between variables difficult. If there are so many data points to plot, overplotting happens, causing various data points to overlap. Plotting the diagram requires a few quick steps.Ī few limitations that have been found with the use of scatter plots are below: It is possible to calculate the data flow's range, i.e., the maximum and minimum values. It's the most effective way to demonstrate a non-linear sequence. It depicts the relation between two variables. The following are some of the benefits of using a scatter plot: Part 3: Benefits and Limitations of a Scatter Plot


 0 kommentar(er)
0 kommentar(er)
